IWK organisiert das erste MAPPER Symposium
26.06.2025
©shutterstock / Hybrid Graphics, bearb. von mdw
Das Institut für musikalische Akustik – Wiener Klangstil organisiert das erste MAPPER – A Symposium on Music Acoustics for Performance Preparation and the Engagement with Repertoire in Live-Electronics. Das Symposium findet am 1. und 2. Juli 2025 auf dem Campus der mdw im Klangtheater (Future Art Lab) statt. Das Symposium bringt Expert_innen aus Asien, Südamerika, Europa und Afrika zusammen und fördert den Wissensaustausch durch eine Vielzahl von Vortragsperformances, Gruppendiskussionen und Live-Präsentationen.
Kontakt: stojak@mdw.ac.at
Semesterende 2025
25.06.2025
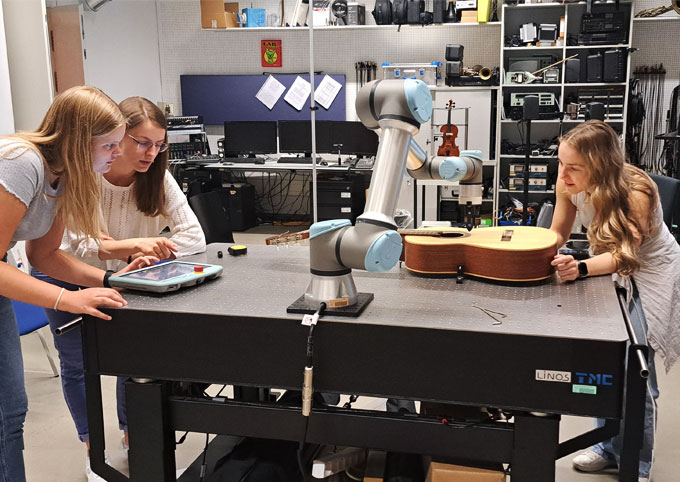
Das Institut für musikalische Akustik – Wiener Klangstil blickt auf ein erfolgreiches Studienjahr 2024/25 zurück. Alle Lehrenden des Instituts hatten viel Freude daran, unseren Studierenden Themen aus dem Bereich der musikalischen Akustik in den unterschiedlichsten Lehrveranstaltungen näherzubringen. So auch in der Lehrveranstaltung „Musikwissenschaftliches Praktikum”, in der die Studierenden zum Abschluss unseren Industrieroboter selbstständig programmiert haben (hier ein kurzer Einblick).
Wir wünschen allen Studierenden einen erholsamen Sommer und freuen uns auf das nächste Studienjahr!

Projektbewilligung im Rahmen der CLARIAH-AT-Mitgliedschaft
23.05.2025
Das Institut für musikalische Akustik – Wiener Klangstil freut sich bekanntzugeben, dass die Einreichung des Projekts "Ringvorlesung Musikwisssenschaft & Digital Humanities" in Kooperation mit dem Institut für Musikwissenschaft und Interpretationsforschung im Rahmen der kürzlich abgeschlossenen CLARIAH-AT-Mitgliedschaft der mdw erfolgreich war. Das Projekt wurde von Melanie Unseld (Institut für Musikwissenschaft und Interpretationsforschung) und Werner Goebl (Institut für musikalische Akustik – Wiener Klangstil) konzipiert und eingereicht. Es wird einen wichtigen Beitrag zur Weiterentwicklung der Digital Humanities an der mdw leisten. Im Zuge dessen ist ab dem Sommersemester 2026 die Einführung einer Ringvorlesung geplant.
Kontaktpersonen: David Morrison-Weigl und Chanda VanderHart
SAR Konferenzbesuch
13.05.2025
Die „16th SAR International Conference on Artistic Research” fand vom 7. bis 9. Mai an der Universität Porto statt. Sie brachte führende Praktiker_innen, Wissenschaftler_innen und politische Entscheidungsträger_innen zusammen, um herausragende künstlerische Forschungsprojekte vorzustellen und kritische Debatten zu zentralen Themen zu führen. Im Rahmen der diesjährigen Konferenz präsentierte unser Institutsmitglied Dustin Zorn seine Forschung über „Resonating with live-electronic ghost tones”.

IWK besucht die Sammlung alter Musikinstrumente im KHM
27.02.2025
Die Mitglieder des Instituts für musikalische Akustik – Wiener Klangstil wurden zu einer exklusiven Führung durch die Sammlung alter Musikinstrumente im Kunsthistorischen Museum Wien eingeladen. Die Führung durch den neuen Direktor des Museums, Dr. Sebastian Kirsch, ermöglichte einen einzigartigen Einblick in die beeindruckende Sammlung alter Musikinstrumente und die Restaurierungswerkstatt für Musikinstrumente. Der Besuch diente dem fachlichen Austausch zwischen der Forschung im Bereich der musikalischen Akustik und der Museumskuration.

Music Encoding News
22.01.2025
Wir freuen uns, zwei aktuelle Entwicklungen in der Welt der Musikcodierung bekannt zu geben, die von den Forschenden des IWK mitgestaltet wurden:
mei-friend v.1.2 wurde veröffentlicht, mit wichtigen neuen Funktionen, darunter eine spezielle Schnittstelle für editorial markup, die von Anna Plaksin an der Universität Paderborn/KreativInstitut Ostwestfalen-Lippe in Zusammenarbeit mit Werner Goebl und David M. Weigl (IWK) beigesteuert wurde. Bitte beachten Sie die Dokumentation der neuen Funktionen (https://mei-friend.github.io/docs/advanced/markup/), die von Sophie Stremel (Paderborn) erstellt wurde.
MEI v5.1 wurde veröffentlicht und bietet eine verbesserte Unterstützung für die Kodierung von Saitentabulaturen, einschließlich deutscher Lautentabulaturen. Diese können nun dank der von Paul Overell eingebrachten Verbesserungen mit Verovio ab Version 4.5 gerendert werden. Die Unterstützung für deutsche Lautentabulaturen ist das Ergebnis einer Zusammenarbeit zwischen dem E-LAUTE-Projekt (https://e-laute.info) und der MEI Tablature Interest Group, mit Beiträgen von David Lewis (University of Oxford/Goldsmiths University of London), Reinier de Valk (University of Vienna), Olja Janjuš (Ludwig-Maximilians-Universität München/mdw) und David M. Weigl (IWK), aufbauend auf umfangreichen Vorarbeiten der MEI-Gemeinschaft.

First Prize Student Award - ASA Meeting
17.01.2025
Unser Projektmitarbeiter und PhD Student Titas Lasickas hat den First Prize Student Award beim Fall 2024 ASA Meeting gewonnen. Sein Paper mit dem Titel 2aMU3. Flow measurements in a bass recorder using particle image velocimetry wurde von der Jury als herausragend bewertet. Wir gratulieren ihm ganz herzlich zu diesem Erfolg!
Proceedings von der Csound Konferenz veröffentlicht
19.12.2024
Am Institut für musikalische Akustik – Wiener Klangstil wurden die Proceedings der siebten Csound-Konferenz veröffentlicht. Die neuesten Proceedings der Csound-Konferenz behandeln zentrale Themen wie Sound Synthesis and Web Apps, GUIs and Skills in Live-Electronics, Csound Expansion und Integrated Csound. Die Beiträge bieten einen Einblick in innovative Ansätze zur Klangerzeugung und Benutzeroberflächen für Live-Elektronik. Besondere Aufmerksamkeit gilt der Weiterentwicklung von Csound.

Forschungsaustausch mit dem IEM aus Graz
26.11.2024
Das Institut für musikalische Akustik – Wiener Klangstil (IWK) der mdw und das Institut für Elektronische Musik und Akustik – IEM der Universität für Musik und darstellende Kunst Graz haben einen intensiven Forschungsaustausch organisiert. Im Zentrum des Treffens standen die Präsentation aktueller Forschungsprojekte, ein Überblick über die jeweiligen wissenschaftlichen Schwerpunkte sowie die Verknüpfung von Forschung und Lehre. Die inspirierenden Beiträge der Forschenden des IEM und IWK trugen maßgeblich dazu bei, den Austausch lebendig und erkenntnisreich zu gestalten. Besonders spannend war die gemeinsame Besichtigung der Räumlichkeiten des IWK sowie des hochmodernen Future Art Labs an der mdw.